Unperceived Objects: An Illustration

Click here and press the right key for the next slide (or swipe left)

also ...

Press the left key to go backwards (or swipe right)

Press n to toggle whether notes are shown (or add '?notes' to the url before the #)

Press m or double tap to slide thumbnails (menu)

Press ? at any time to show the keyboard shortcuts

If you've seen the outline of lectures, you'll know that my idea is to organise the lectures by domains of knowledge. As we will see, how we first come to know things about colours, say, isn't quite the same as how we first come to know things about minds. But there is one very general point we can make: in all these domains, we will face Davidson's challenge, the challenge of explaining what is inbetween mindless behaviour and thought.

plan

objects

causes

colours

words

non-verbal communications

minds

actions

Let me preview how Davidson's challenge arises in the case of objects.

When do humans first come to know facts about the locations of objects they are not perceiving?

A famous study by Renee Baillargeon and her collaborators provides evidence that humans can represent unperceived objects from around four months of age or earlier. This is called the 'drawbridge study'

What you are about to see are the test events from Experiment 1 of Baillargeon et al's 1987 study. You're looking at them from the side whereas the subjects, four-month olds, were looking at them from the front (which is to your right).

In showing you these test events, I need to explain the method used in this experiment, \emph{habituation}; this is a method we will encounter repeatedly so it's good to understand how it is supposed to work.

%glossary: habituation

What you see here is a barrier rotating through 180 degrees. Infants were habituated to this; that is, they were shown it repeatedly until it no longer held their interest. The first time they're shown this, they might spend 60 seconds looking at it, which is a long time for an infant; but after, say, five demonstrations, they'd only be looking at it for around 10 seconds. That is, they are habituated to this display.

\begin{center} \citealp{baillargeon:1987_object} figure 1 \end{center}

Baillargeon (1987, figure 1)

Now there is a very small change to the display. The display is just as before, except for before the drawbridge moves an object is placed behind it. There are then two different things that could happen. One is that the drawbridge moves exactly as before, rotating a full 180 degrees. This is called the 'impossible event'. The other is that the drawbridge now rotates for 120 degrees, which is the 'possible event'. In no case is the object visible after the drawbridge has started moving. We want to know which events infants find more novel. If they are unable to know facts about the locations of unperceived objects, then they should find the 'possible event' more novel than the 'impossible event' because it is more different from the event they have been habituated to. On the other hand, if infants are able know facts about the locations of unperceived objects, they should find the impossible event more novel than the possible event because, well, it's impossible.

To find out what infants find more interesting, they are divided into two groups. One group sees the impossible event, the other the possible event. The experimenters measure how long the infants look at these events, which is the measure of their dishabituation. The background assumptions are that looking longer indicates more interest, and that interest is driven by novelty.

In the control condition, 'The habituation event was exactly the same as the impossible event, except that the yellow box was absent.' (Baillargeon et al 1985, 200)

These are the results from Experiment 1 of Baillargeon et al's 1987 study.

This experiment provides evidence that infants know that the object is behind the barrier even when they can't see it, for their having such knowledge would explain why they appear surprised by the impossible event.

\begin{center} \citealp{baillargeon:1987_object} figure 2 \end{center}

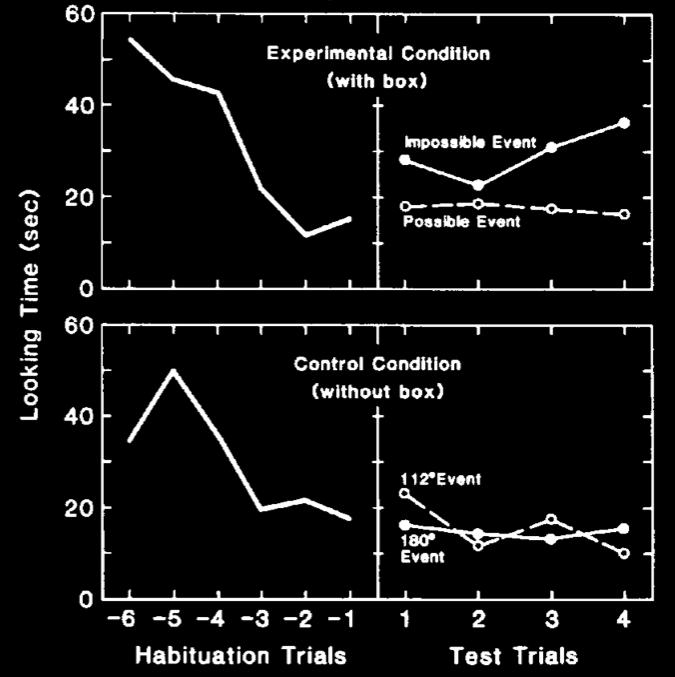
source: Baillargeon et al (1987, figure 2)

Here you can see, reassuringly, that the effect is not present in the control condition where the box is absent.

Some have been critical of the methods used in this experiment. But not everything hangs on this experiment. Fortunately there are at least a hundred further experiments which provide evidence pointing in the same direction. Later we'll look at this is more detail.

When do humans first come to know facts about the locations of objects they are not perceiving?

look: by 4 months of age or earlier (Baillargeon 1987).

This result has been widely replicated, and it coheres with a large body of research we shall explore later.

look: by around 2.5 months of age or earlier (Aguiar & Baillargeon 1999, Experiment 2)

By using more sensitive methods, \citet{Aguiar:1999jq} even demonstrated competence in a group of 2.5 month old infants.

So far so good, but there is a problem ... What happens if instead of measuring how infants look, we measure how they reach?

\citet{Shinskey:2001fk} did just this. Here you can see their appratus, which is quite similar to what \citet{baillargeon:1987_object} used. They had a screen that infants could pull forwards to get to an object that was sometimes hidden behind it. They made two comparisons. First, were infants more likely to pull the screen forwards when an object was placed behind it? Second, were how did infants' performance compare when the barrier was not opaque but transparent?

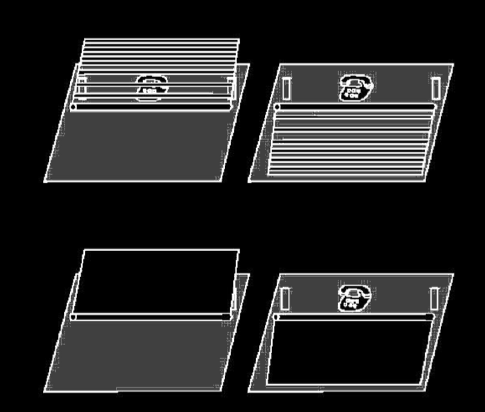
Shinskey and Munakata 2001, figure 1

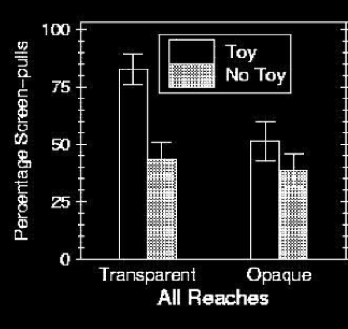
Here are their results with 7-month old infants.

Shinskey and Munakata 2001, figure 2

We are interested in whether infants were more likely to pull the screen forwards when the object was present than when it was absent. Since infants wanted the toy, if they knew it was behind the barrier they should have pulled forward the barrier more often when the toy was behind it. This is exactly what they did when the barrier was transparent. But look what happens when the barrier is opaque, so that the toy is not visible to infants when they have to prepare the pulling action: they no longer pull the barrier more often when the toy was behind it.

This is good evidence that 7 month olds do not know facts about the locations of objects they cannot perceive. And this is not isolated evidence; for example, \citet{moore:2008_factors} use a different methods also involving manual search to provide converging evidence for this conclusion. But now we have a problem ...

When do humans first come to know facts about the locations of objects they are not perceiving?

look: by 4 months of age or earlier (Baillargeon 1987).

look: by around 2.5 months of age or earlier(Aguiar & Baillargeon 1999, Experiment 2)

search: not until after 7 months of age (Shinskey & Munakata 2001)

The evidence appears to be contradictory.

By measuring looking actions, we find infants can distinguish situations in ways that indicate they do know facts about the locations of particular unperceived objects.

But when measuring retrieval or searching actions, we find infants cannot distinguish these situations; this indicates that they cannot know this.

You might hope there would be a simple solution. Perhaps, for example, infants have difficulties reaching that mask their real knowledge of the facts about unperceived objects' locations. But As Jeanne Shinskey, one of the researchers most dedicated to this issue says,

‘action demands are not the only cause of failures on occlusion tasks’ \citep[p.\ 291]{shinskey:2012_disappearing}.

Many such explanations have been tried because many researchers have been puzzled by this; \citet{Meltzoff:1998wp} go as far as to call it a paradox (the 'paradox of early permanence'). No explanation positing extraneous task requirements, such as difficulties performing an the actions required, has yet succeeded.

This is a discrepancy between two types of measure; one involves looking, other other searching. We find this pattern--discrepant findings pointing to opposite conclusions about what infants and adults know--in many different domains.

As \citet[p.\ 994]{charles:2009_object} put it, these findings are ‘the tip of an iceberg’.

‘violation-of-expectation experiments, using looking-time measures, suggested that infants have object permanence in occlusion conditions; but simplified-search studies confirm that infants fail to reach towards occluded objects, suggesting that infants do not have object permanence in occlusion conditions. This discrepancy, however, is only the tip of the iceberg. Results of studies attempting to measure infants’ cognitive abilities using reaching measures often contradict results gained while using looking-time measures.’ \citep[p.\ 994]{charles:2009_object}

What is the problem?

You might be wondering whether there's a philosophical problem here. Science is a messy business and you get conflicting results all the time. But this particular pattern of conflicting results is extremely interesting philosophically. It shows that we cannot say that, at, say, five months of age, infants know facts about the locations of particular unperceived objects. We cannot say this because doing so generates predictions which are clearly false (predictions about where they will search for an unperceived object). But it also shows that we cannot say that they have no sense at all concerning facts about the locations of particular unperceived objects. We cannot say this because of the competence they manifest in distinguishing possible from impossible events.

The problem, then, is that understanding the origins of knowledge requires us to identify something inbetween knowledge and its absence, something that is like knowledge in some respects but falls short of it in others. This is an instance of Davidson's challenge ...

Uncomplicated Account of Knowledge

Think of the spider as the object behind Baillargeon’s drawbridge and behind Shinskey and Munakata’s screens.

Can Wy (the infant) act for the reason that there is an object behind the drawbridge?

Yes: she looks longer when the drawbridge rotates 180 degrees for the reason that there is an object behind it.

No: if she could act for the reason that there is an object behind behind Shinskey and Munakata’s screens, then she would search for that object.

So Wy is awkward. She can do some things for the reason that there is an object behind a screen (e.g. look longer), but she cannot do other things for this reason. This is not allowed for by the Uncomplicated Account of Minds and Actions.

We can’t say Wy belives that there is an object behind the screen. In the absence of obstacles such as time pressure, distraction, motivations to be irrational, self-deception or exhaustion, humans will act in ways that are approximately rational given their beliefs and other attitudes.

So the hypothesis that Wy belives that there is an object behind the screen generates the incorrect prediction that she will manipulate the screen to reveal that object. Which she does not.

I’ve suggested that we can’t say that Wy belives that there is an object behind the screen. Yes, puzzlingly, she can perform some actions for the reason that there is an object behind the screen.

So she is not entirely neutral on whether there is an object behind the screen.

This is an objection to the correctness of the Uncomplicated Account of Minds and Actions. It doesn’t work, at least not for infants. (Later I’ll suggest it doesn’t work for adults either, if you look closely enough at what they’re doing.)

\textit{Object permanence}: the ability to know facts about objects you aren't currently perceiving.

Keyboard Shortcuts?

Unperceived Objects: An Illustration

Keyboard Shortcuts`?`